Hands-on math!

Washington State Math Olympiad
Hints and Solutions
2007 Grade 5 Geometry
Problem
Hint
1) A square is outlined in string and has an area of 64 square units. If you take the string to make a regular octagon that has the same perimeter as the square, how long is one side of the octagon?

 1. Compute the length of one side of the square = ____ units.
2. Compute the perimeter of the square = ____ units.
3. Divide this by 8 to get the length of one side of the octagon = _____ units.
2) The quadrilateral ABCD has parallel sides AB and DC. Side AD is perpendicular to side AB. What is the sum of the angles at B and C?

 1. If side DC is parallel to side AB and also perpendicular to it, then the two angles BAD and ADC are _____ each.
2. Since a quadrilateral's angles all add up to ____,
    the angles at B and C add up to ____ degrees.
3) A tangram puzzle has 7 shapes that can be put together to make a square as shown. If the side of the large square measures 4 inches, what is the area of the small square? Use the Pythagorean theorem.
1. If you can find the length of a side of the small square you can determine it's area.
2. The length of a side of the small square is half the length of the adjacent side (call it "L") of the large right triangle whose hypotenuse is 4 inches (the outer edge length).
3. Since the other 2 sides of the large triangle are the same, determine the length of one of those sides:
    Then L2 + L2 = 42.
4. Solve this for L = _____
5. Take half of that = _____
6. The area of the small square is _____.

Problem
Hint
4) Right triangle ABC shown in the figure has a right angle at B and a 50oangle at A. Reflect triangle ABC over side BC to create a new larger shape made up of two triangles. What's the measure of the new big angle at vertex C?
 1. The angle at C is complementary with the angle at A and is _____ degrees.
2. If you reflect around line BC, then the angle at C is doubled = _____ degrees.
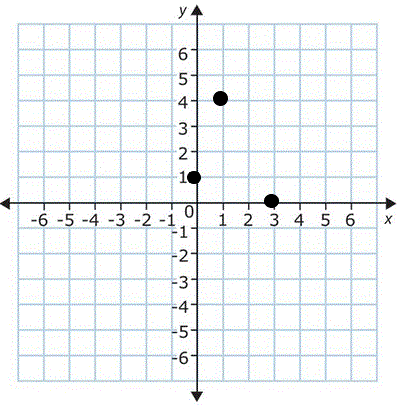
5) Rita wants to draw a square on a coordinate graph. The square is rotated a little bit around its center. She puts one vertex at (3,0), one vertex at (0, 1), and one vertex at (1, 4). Where will the last vertex go to complete the square? Use the coordinate graph provided. 1. Plot the 3 points on the graph.
2. Determine the offset of the second point (0,1) from the first (3,0). This is going from point 2 to point 1. The offset is (___,___).
3. Apply this offset from the third point (1,4) to get the coordinates of the 4th point = (___,___).