Hands-on math!

Washington State Math Olympiad
Hints and Solutions
2007 Grade 6 Geometry
Problem
Solution
1) A tangram puzzle has 7 shapes that can be put together to make a square as shown. If the side of the large square measures 4 inches, what is the area of the small square? 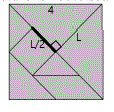Use the Pythagorean theorem.
1. If you can find the length of a side of the small square you can determine it's area.
2. The length of a side of the small square is half the length of the adjacent side (call it "L") of the large right triangle whose hypotenuse is 4 inches (the outer edge length).
3. Since the other 2 sides of the large triangle are the same, determine the length of one of those sides:
    Then L2 + L2 = 42.
4. Solve this for L =
    2L2 = 16
    L2 = 8
    L = √8
5. Take half of that = √8 / 2
6. The area of the small square is (√8 )2 / 4 =
    8/4 = 2 sq. in.
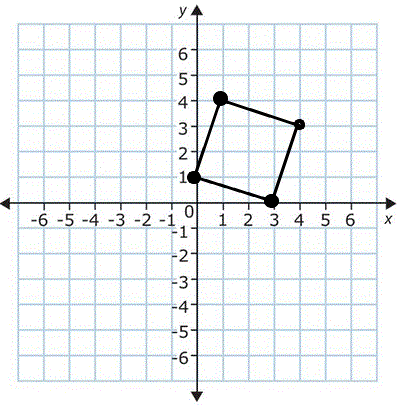2) Rita wants to draw a square on a coordinate graph that's rotated a little bit around its center. She puts one vertex at (2, -1), one vertex at (-1, 0), and one vertex at (0, 3). Where will the last vertex go to complete the square? Use the coordinate graph provided. 1. Plot the 3 points on the graph.
2. Determine the offset of the second point (0,1) from the first (3,0). This is going from point 2 to point 1.
    The offset is (3,-1).
3. Apply this offset from the third point (1,4) to get the coordinates of the 4th point =
    (1 + 3 = 4,4 - 1 = 3) = (4,3)

Problem
Solution
3) Which of the following nets will correctly fold to make a triangular prism with one pointed tetrahedron side? (Think of a pointed three sided stake.)
 1. Visualize folding these flat shapes into the triangular prism with the tetrahedron end.
2. Some of them will have shapes that overlap.
3. The ones that fold correctly are b & c
4) In triangle ABC shown, AB is perpendicular to CB, and DE is parallel to AC. The angle at vertex A measures 42 degrees. What is the sum of the two angles labeled x and y? 1. The angle at C is complementary with the angle at A and is 48 degrees.
2. Since C and A are 2 angles of the ADEC trapezoid, the other 2 angles add with these 2 to make 360 - 90 = 270 degrees.
3. x + y = 270 degrees.

5) A square is enlarged so as to increase its area by 300%. What is the ratio of the new side length to the old side length?

 Careful! Tricky terminology!
1. Assume the original square had a side length of 1
2. Then an increase of 100% in its area
    would mean an area of 2 which is an
    increase in the side length to √2
3. An increase of 200% in its area would mean
    an area of 3 which is an increase
    in the side length to √3
4. Carry this logic forward to get the side length for an increase of 300%. = √4 = 2
5. Express this as the ratio of this length to the original side length = 2/1 or 2:1