Hands-on math!

Washington State Math Olympiad
Hints and Solutions
2008 Grade 5 Geometry
Problem
1) A pinwheel is made of four identical shapes each with a different color arranged counterclockwise in color order red, blue, green, and gold. They are evenly spaced. The wind spins the pinwheel counterclockwise, and when it stops, the red shape is now where the blue shape used to be. If the pinwheel went through two complete rotations before red ended up where blue used to be, how many total degrees did the red shape travel?
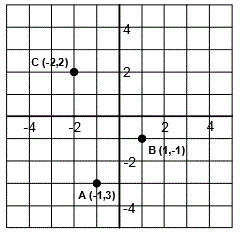
2) John is trying to make a rectangle on the given coordinate grid. He has already labeled the point A at (-1, 3), the point B at (1, -1), and point C at (-2, 2). Where should he plot point D to complete the rectangle?

Problem
3) Toleen's math teacher makes a model of a solid rectangular prism out of white one centimeter cubes to explore surface area and volume. She paints the entire outside surface red. If the dimensions of the prism are 3 cm by 4 cm by 6 cm, how many of the cubes have exactly two sides painted red?
4) In Latoya's class they are cutting out snowflakes from square paper folded in half and them in half again to make a folded square. Latoya cuts along the three lines shown in the picture of the folded square. When she unfolds her paper what shape will fall out of her square?
5) In right triangle AMC (see diagram) triangles ANP and PLC are congruent. MNPL is a square and segment LC has length 2 cm. What is the perimeter of the square MNPL?