| Problem |
Solution |
1) It costs $50.00 each day to run a small pudding factory. It costs 25 cents to make a pudding cup. How many
pudding cups can be made in that day if the factory has $516.00?
|
Let N = number of pudding cups
Then N = ($516 - $50)/$0.25
Solve for N = 466/0.25 = 1864 pudding cups
|
|
2) Two ice cream men are racing to the park along the same route. Ice cream man Chandler is going 24
meters per minute. Ice cream man Joey is going 3600 centimeters in one minute. Chandler gets to the park
in 4 minutes. If they started from the same point, how long will it take Joey to get to the park? Express
your answer in minutes and seconds. |
1. Convert Joey's rate to meters/minute =
3600 cm/100 min = 36 m/min
2. Compute the distance Chandler went in 4 minutes =
24 x 4 = 96 meters.
3. Divide this distance by Joey's rate to get Joey's time =
96/36 = 2.66667 minutes.
4. Convert this to minutes and seconds = 2 min 40 sec
|
3) Jordan's backyard is 1300 square feet. She is willing to devote 12% of her yard to a trampoline. What
would be the diameter of the largest circular trampoline that would satisfy that? Circular trampolines only
come in whole number diameters. Use 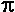 = 3.14 = 3.14 |
1. Compute 12% of the backyard's area =
.12 x 1300 = 156 sq. ft.
2. Use this area to find the radius of the trampoline =
3.14 x R2 = 156
R2 = 156 / 3.14 = 49.68
R = √49.68 = 7.048
D = 2 x 7.048 = 14.0968
3. Round down to the next whole foot = 14 ft.
|