Hands-on math!

Washington State Math Olympiad
Hints and Solutions
2011 Grade 5 Geometry
Problem
Hint

1) Samantha is playing with 100 wooden cubes. She first makes the biggest cube possible out of the 100 cubes. After she makes this cube, there are some small cubes left over. What is the width of the largest size cube she can make with the remaining cubes? 		1. The largest cube she can make with 100 small cubes is
    ___ x ___ x ___ = ____ cubes.
2. Subtract this number of cubes from 100 =
    ____ small cubes left.
3. With ____ cubes left, she can make a
    ___ x ___ x ___ cube.
4. This is ____ cubes on each side.

2) A quadrilateral has 4 lines of symmetry. Three of its vertices are (1,3), (7, 1) and (9, 7). What is the fourth vertex?
1. Plot the 3 points on the provided graph.
2. A quadrilateral with 4 lines of symmetry is a ______.
so now you must find the location of the 4th point that makes a _______.
3. Find the offset from the 2nd point (7,1) to the 3rd point (9,7). Offset = (___,___). 4. Apply this offset to the 1st point (1,3) to get the 4th point = (___,___).

Problem
Hint

3) The area of a rectangle is 48 square feet. The lengths of the sides are whole numbers of feet. What is the largest perimeter the rectangle can have? 		Make a table (L is the length and W is the width):
    L        W     PerimeterArea
     48
   48
   48
   48
   48
Find the combination of length and width that results in the largest perimeter = ______.

4) Ace, Berrit, and Calynn have a loop of string that measures 12 feet around. They each hold it with one hand and pull it taut to make a scalene triangle (none of the sides have the same length). After experimenting with different triangles they realize there is a range of values for the longest side. What are the low and high numbers for the range? Express your answer using inequalities with L representing the length of the longest side. 		1. If Ace and Berrit stretch the string taut with Calynn not holding it, the doubled string length is ___ feet.
2. Suppose Calynn holds the string in the middle and makes an equilateral triangle 4 feet on a side. This is not scalene, but if she moves even an inch to one side, then she has created a triangle with these sides:
  1. 3 ft 11 inches
  2. 4 ft 1 inch
  3. 4 ft
3. From these two pieces of information you should be able to determine the low and high numbers for the longest side.
4. The longest side must be more than ____ feet and less than ____ feet. The inequality is:
lower limit _____ < L < upper limit ____.
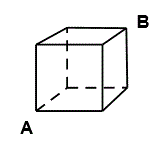
5) The length of the shortest trip from A to B, along the edges of the cube, is 3 edge lengths. How many different 3-edge trips are there from A to B? 1. From A how many paths can you take? _____
2. After you have taken one of these paths, how many can you take from there, assuming you don't go backward? ____
3. From that second point, how many ways are there to get to point B? _____
4. Multiply these together to get the total number of paths = ____.