1) If this pattern continues, in which column will 500 appear?
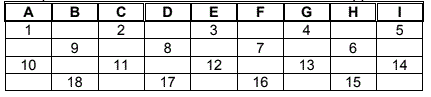
|
This problem involves arithmetic sequences.
1. Notice that in each column the next number is the previous number + 9.
Don't worry about the blank spaces.
2. Compute which element of the sequence that 500 will occur in by dividing it by 9 = _____th element.
If this is not an even number, round up = _____ th element.
3. The formula for finding the nth element of an arithmetic sequence is:
An = A1 + D x (n - 1)
where D is the amount added to each element
4. Using this formula (your An is 500), find the column with the appropriate starting number that makes the ____th element 500.
5. That column is _____
|
2) Jimmy and Carlos are at a meeting with eight other people. They are all hungry, but there are only seven bagels. Jimmy divides each bagel in half and gives each person a half of a bagel. He takes the remaining pieces and keeps dividing them all in half until he can again give everyone an equal size piece. He repeats this again with the remaining pieces he now has until everyone has gotten 3 of the same size pieces, a large piece, a medium piece and a small piece. If Jimmy takes his fair share and any remaining pieces, what fraction of the bagels does Jimmy get? Express your answer to the nearest 1/16th of
a bagel. |
1. There are 7 bagels making ___ half-bagels.
2. After passing out 10 half-bagels, there are ___ half-bagels left which he cuts in half to make ____ quarter-bagels
3. After passing these out there is ___ quarter-bagels left, which he cuts in half to make _____ eighth-bagels
4. Keep cutting until you have at least 7 pieces to distribute and then the remainder will be ______
5. This leaves ____ (what size) bagel left, which is his.
6. So, each person got ______________________ leaving ____ left, which is his.
7. So, Jimmy got what everyone else got: _________________
plus _____ which sums to ______ of a bagel.
|