|
Washington State Math Olympiad
Hints and Solutions
2016 Grade 6 Geometry
|
 |
| Problem |
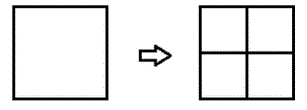1) A square can be split to make four smaller
squares of equal sizes to completely fill the
original square as shown. How would you split up
the original square into six smaller squares that
completely fill the original square without any
overlaps of the smaller squares?
(The squares do
not all need to be the same size.)
|
2) If the total surface area of a cube is 150 sq. cm., what is its volume?
|
|
3) In rectangle ABCD, X is the midpoint of CD and
Y is the midpoint of AD. What percentage of the
figure does the shaded area represent?
5) How long is segment BC?