Hands-on math!

Washington State Math Olympiad
Hints and Solutions
2014 Grade 6 Geometry
Problem
Solution
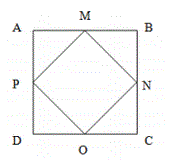1) ABCD and MNOP are squares. M, N, O, and P are at midpoints of the four sides of the square ABCD. If MN is 8 inches, what is the area of the square ABCD? 1.If the points MNOP are midpoints of the sides of ABCD, then the area of MNOP is 1/2 the area of ABCD.
2. The area of MNOP is 8 X 8 = 64 sq. in.
3. The area of ABCD = 2 X 64 = 128 sq. in.
2) Jenny build a pyramid out of sugar cubes to model a Mayan pyramid. Each layer is a solid square of sugar cubes and the pyramid looks the same on all four sides. The bottom layer shows 10 cubes along each side. The next layer shows 9 cubes along each side and so on ending with one cube on top. Jenny paints all the surfaces of the pyramid that are showing. How many cubes have no paint on them? 1. If you lay out a square of cubes, 10 on a side (the bottom layer), then that makes 100 total cubes.
2. The cubes on just this one layer that will not have paint are the ones on the inside, making a square that is 8 cubes on a side.
3. Apply this to the remaining layers to compute the number of cubes that will not have any paint:
    8 x 8 = 64
    7 x 7 = 49
    6 x 6 = 36
    5 x 5 = 25
    4 x 4 = 16
    3 x 3 =  9
    2 x 2 =  4
    1 x 1 =  1
Total: 204 unpainted cubes
3) A cubic shaped fish tank is 40 inches tall. One quarter of the tank is filled with water. A metallic cube is added to the tank that has edge length 30 inches. How much water in cubic inches is needed to fill the tank the rest of the way? 1. Compute the volume of the tank = 40 x 40 x 40 = 64,000 cu. in.
2. Compute the volume of the metallic cube = 27,000 cu. in.
3. Compute the tank volume - the cube volume - the 1/4 of the water in the tank = 64000 - 27000 - 64000/4 = 21,000 cu. in.

Problem
4) A rectangular garden 30 m by 40 m will have a concrete walkway of uniform width all the way around. Find what width of the walkway to the nearest foot so that the area of the walkway equals the area of the garden.
Solution
Method 1: Add up the walkway areas:(see figure to the right)
1. Compute the area of the garden A = 1200 sq. m.
2. Let W = width of the walkway.
3. Then, the area of the walkway is:
    The top and bottom walks: 2 x (40 x W) +
    The 2 side walks: 2 x (30 + 2W) x W = 1200
    80W + 60W + 4W2 = 1200
    140W + 4W2 = 1200 sq. m.
    35W + W2 = 300
    W2 + 35W - 300 = 0
4. This is a quadratic equation (has a square term) and you need to use the quadratic formula to solve this:

    W = - 35 ± √ 35 2 - (4)(1)(-300)      So:
                  2
    W = - 35 ± √1225 +1200      =
                  2
    W = - 35 ± √2425      =
                  2
    W = - 35 ± 49.2     = 7.12 or -42.1
                  2
Since we can't have a negative pathway width, the only answer is: (rounded) 7 ft.
Method 2: Use area subtraction:(see figure to the right)
1. Compute the area of the garden A = 40 x 30 = 1200 sq. m.
2. Let W = width of the walkway.
3. The area of the garden + its walkways =
    (30 + 2W) x (40 + 2W) =
    1200 + 60W + 80W + 4W2
    4W2 + 140W + 1200
4. Set this equal to twice the garden area
(since the garden + walkways = twice the garden area):
    4W2 + 140W + 1200 = 2400
    W2 + 35W + 300 = 600
    W2 + 35W - 300 = 0
5. This is the same quadratic equation as in Method 1,
so the solution is the same:
    W = (rounded) 7 ft.

Problem
Solution
5) The geometry net shown folds to a cube with a missing face. On this figure mark with an x two edges where the final square can be placed so that the net will fold to a cube with no missing faces. 1. Assume the middle face is the one facing you.
2. Then folding the 2 upper ones down, the left one (of the upper 2) becomes the top and the right one the right side of the cube.
3. Folding the left 2 sides in, the upper side (of the left 2 sides) becomes the left face and the lower one the bottom.
4. So the missing face is the back one on the other side of the middle face (the side facing away from you).
5. Find 2 places to put a new square that folds into that back face position.
The places are shown in the figure above(any 2):